Math

If you'd like to go deep into the Montessori math program for children ages 3 to 6+, read our Math Album/Teacher Manual. But, you can start with this overview.

Overview

The mathematical mind has the ability to abstract, reason, investigate, imagine, calculate, measure, and be exact. The mathematical mind is part of the absorbent mind. It develops naturally as the child grows and we typically see an acute interest and capacity for enumeration beginning around age four (sometimes sooner, sometimes later). 

The sensorial materials indirectly prepare the child for work in mathematics because of their innate sequence, order, and progression. Sensorial activities develop the child’s precision, exactness and provide clear, exact mathematical experiences. For example:

• Pink tower (cubes from 1 cm to 10 cm; focuses on order and dimension)
• Brown stair (changing by 1 cm increments; focuses on order and dimension)

By isolating these materials, we lead the child towards abstracting the concepts of cubing and squaring. For these reasons, extensive work with the Montessori sensorial materials should almost always occur before beginning math work. 

The math materials proceed in an enjoyable fashion that leads the child to think logically and to reason. The materials are concrete, structured, rational, and logical. Early materials produce the sensorial impression of each process—the focus is on the process NOT the accuracy of the results. 

We begin with enumeration/counting exercises. We always introduce the concrete first (e.g., the number rods) and then the symbol (e.g., sandpaper numerals) only after the child has adequate experience working with the concrete. This is just as in language we introduce spoken language and phonemic awareness work before beginning the sandpaper letters.

Once they have mastered counting and symbol from zero to ten, we introduce the decimal system to concretize the base ten system. Finally, we sequentially introduce the operations of addition, multiplication, subtraction, and division (both short and long division). All exercises begin with a great deal of activity using concrete materials such as the golden beads. Only after the child has internalized, do we move on to more abstract materials like the stamp game or strip boards. Again, in all of this early work, we are focused on the sensorial impression of the process rather than the accuracy of results. Accuracy naturally refines itself as the child grows into her mathematical mind. This is the same way that spelling naturally refines itself as the child gains experience with reading.

The process of discovering the mathematical truths is a gorgeous experience for the child. We must allow them the freedom and opportunity to arrive at the basic conclusions/discoveries on their own—they will feel like they are the first ones to discover it!

Characteristics of the Materials

Math Lessons

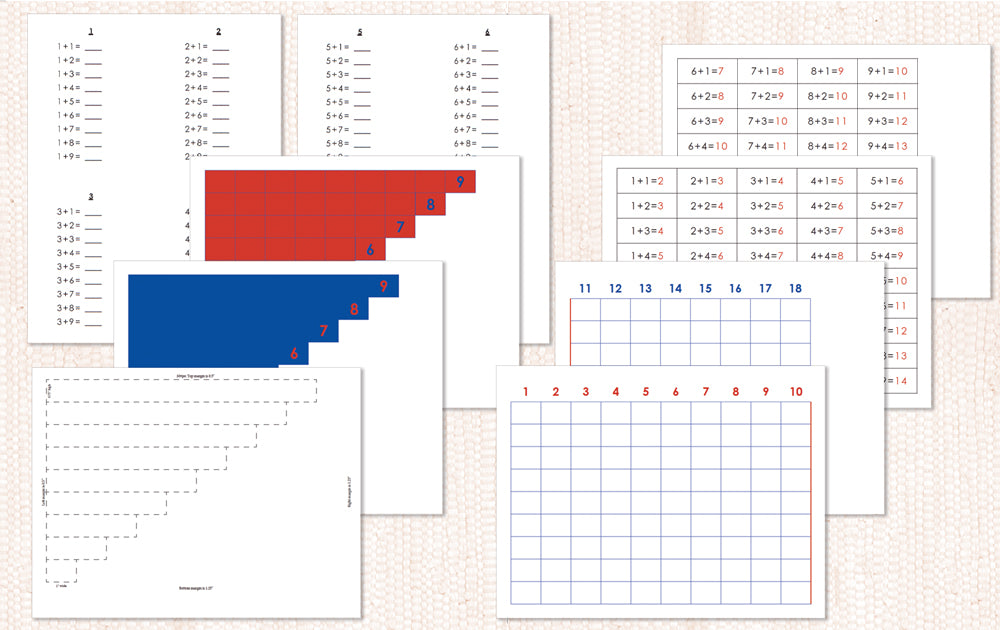
The outline below does not go precisely in linear order...learning is dynamic and many links are made by the child. We always begin with the complete Enumeration 1 to 10 sequence. After that, we simultaneously branch into the Decimal System and Enumeration 10 and above. Once a child has a solid understanding of the decimal system, we move to the operations and present them in a specific order: (1) Addition, (2) Multiplication, (3), Subtraction, (4) Division (short and then long).

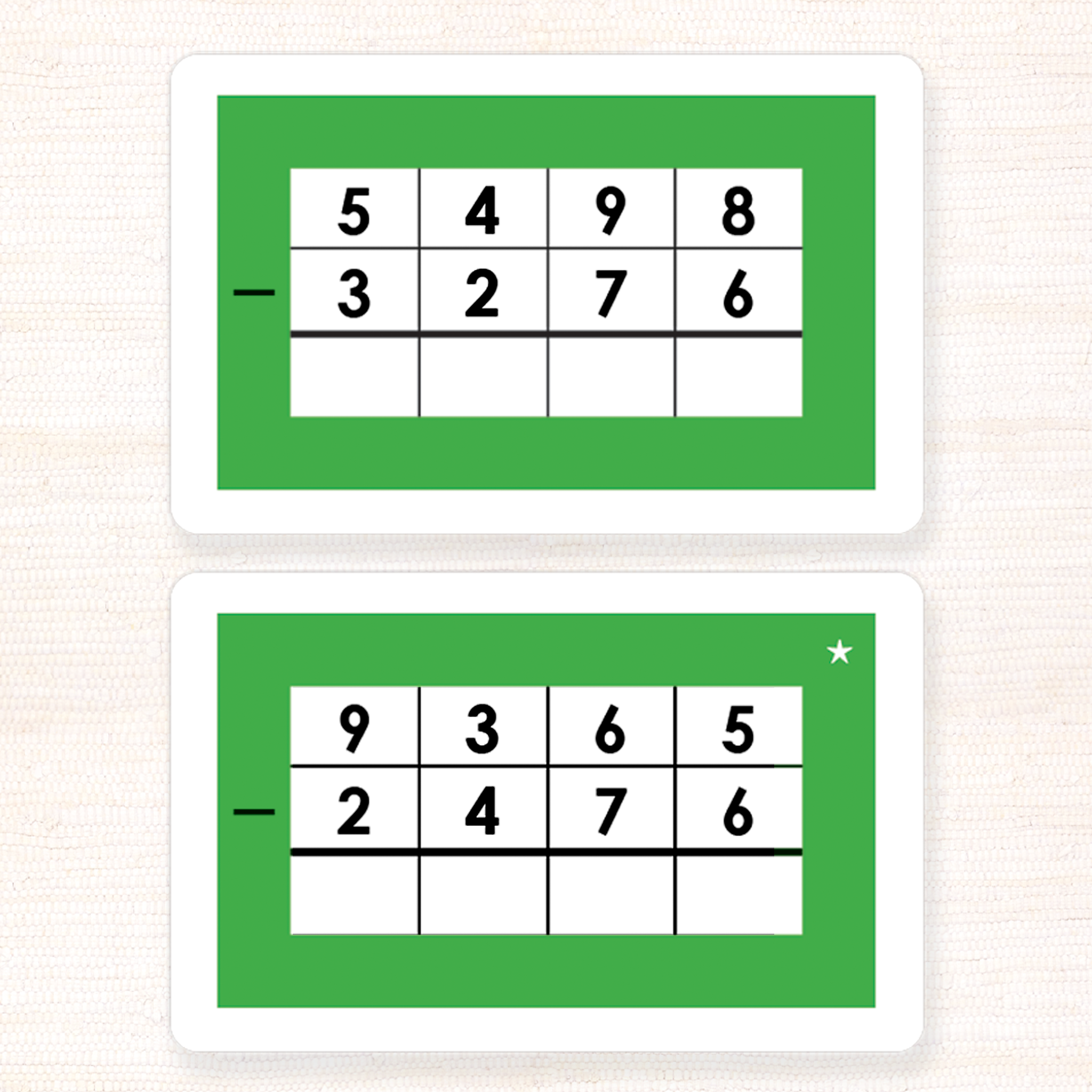
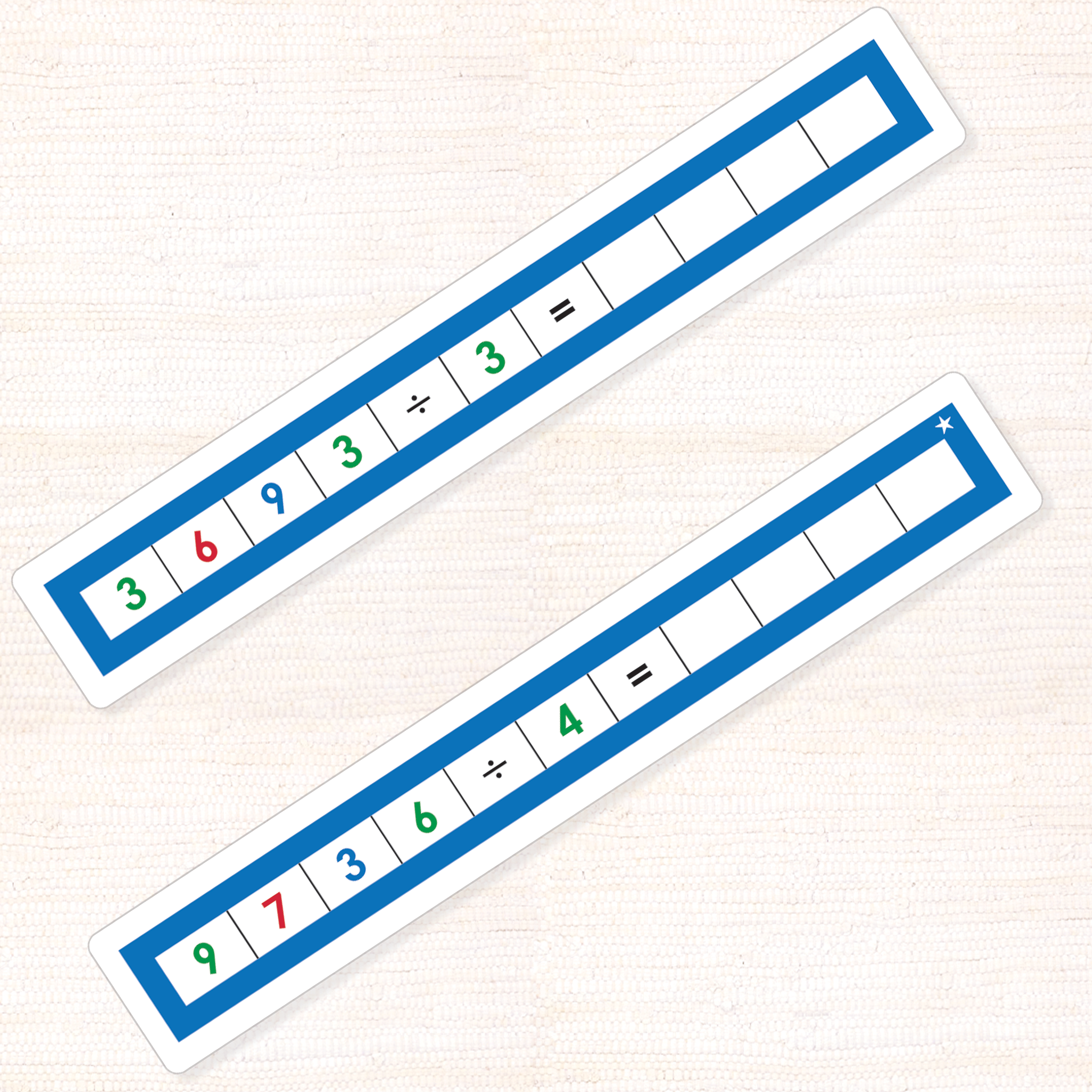
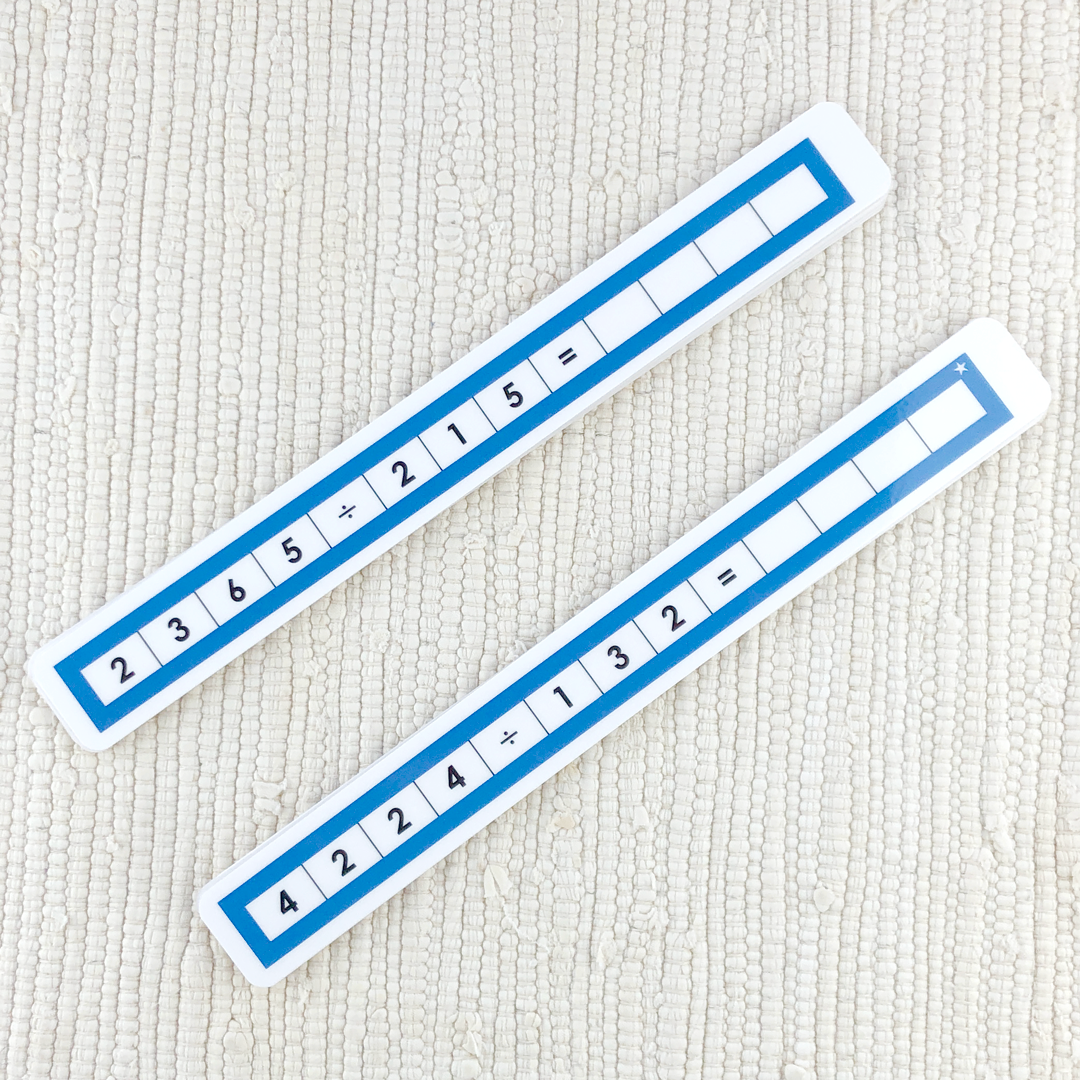
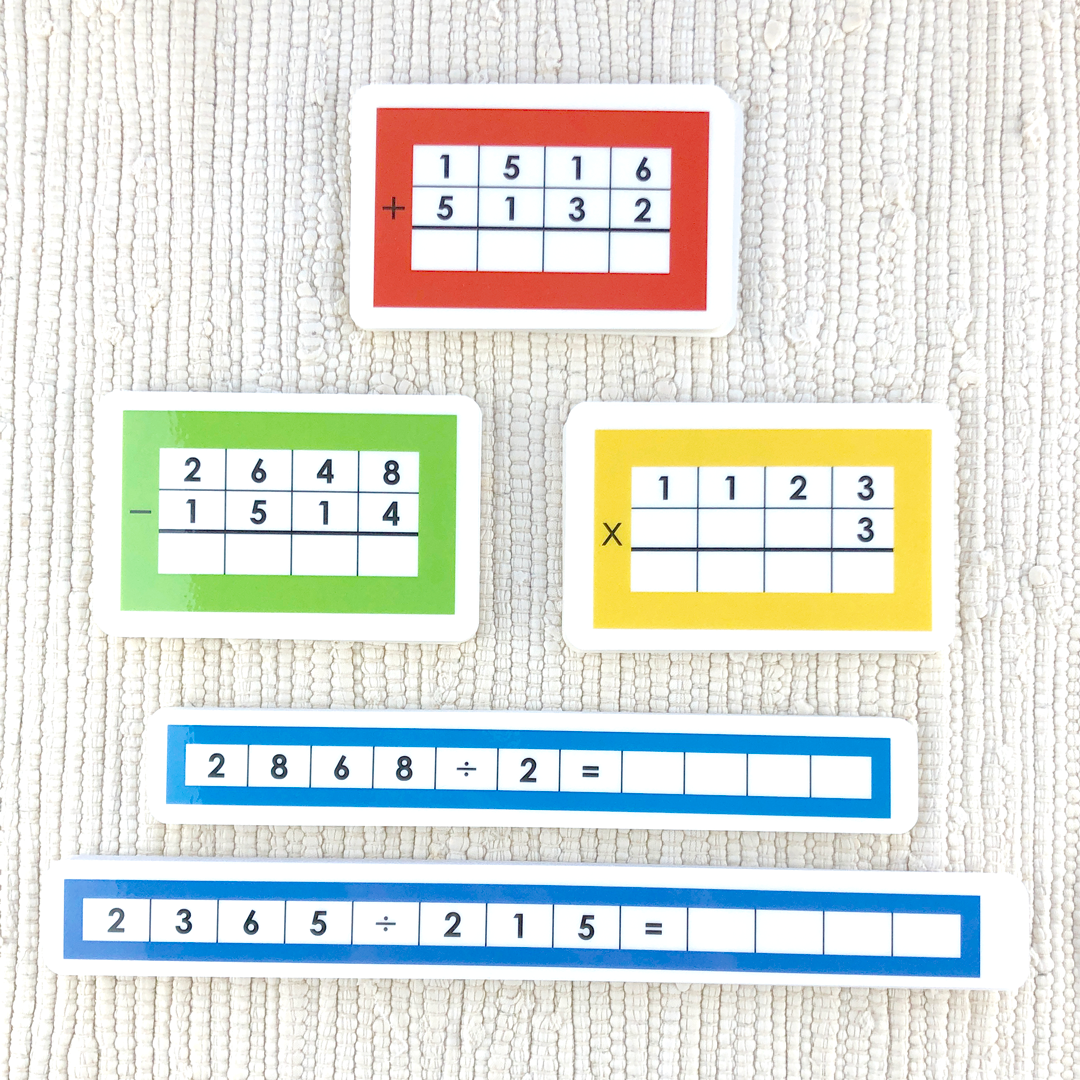
The initial work for all operations happens with base 10 manipulatives. The focus is on the process rather than on precision at this stage. Montessori classrooms use the golden beads (often called the bank game in prek-K classrooms). Static equations are introduced first in order to isolate the operations' process without complicating it with borrowing/exchanges. We move on to dynamic equations only once the operations' concept is clear to the child (addition means putting it all together, multiplication means putting the same thing together so many times, subtraction means taking something away, division means taking the same thing away so many times). But, we don't have to do static equations for all operations before moving into dynamic ones. For this, we follow the child. For example, some children may be doing dynamic addition and multiplication equations before we introduce subtraction. But we must not overlook both short and long division! 

So, the following gives you a general idea of how the materials/activities progress but it does not present the nuance of how this may actually unfold for a given child. In Montessori, we always follow the child and use the lessons as a structure to guide our decisions around the observable needs, interests, and capacity of the individual before us.

Enumeration 1 to 10

• Red rods (indirect preparation; once they can align these easily, it is our sign to introduce the number rods)
• Number rods (concrete)
• Sandpaper numbers (symbol)
• Number rods & cards (concrete & symbol)
• Spindle Box (concrete & symbol)
• Cards and counters (concrete & symbol)
• Memory game of numbers (concrete & symbol)

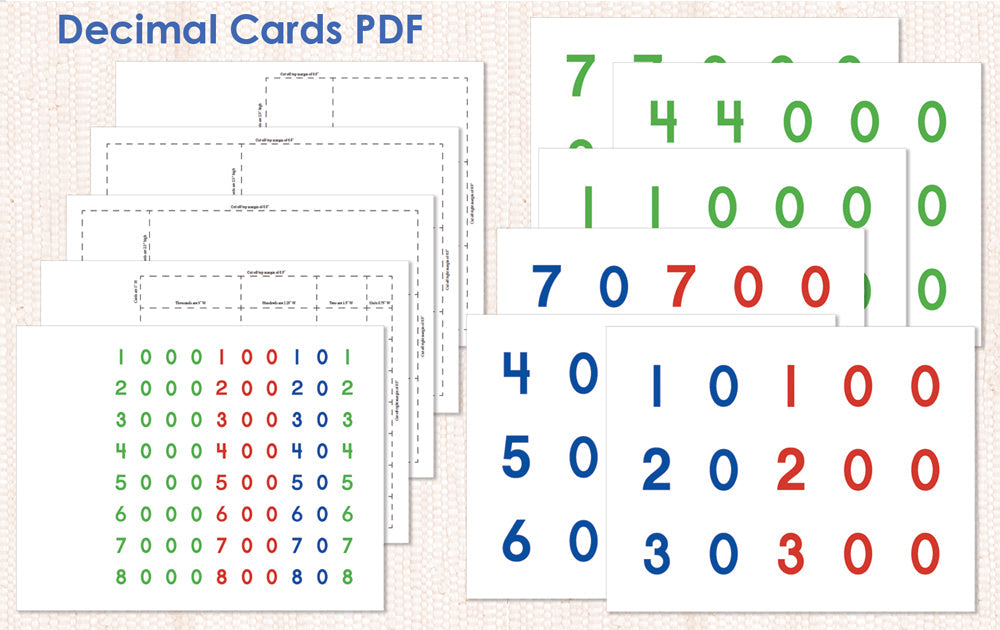
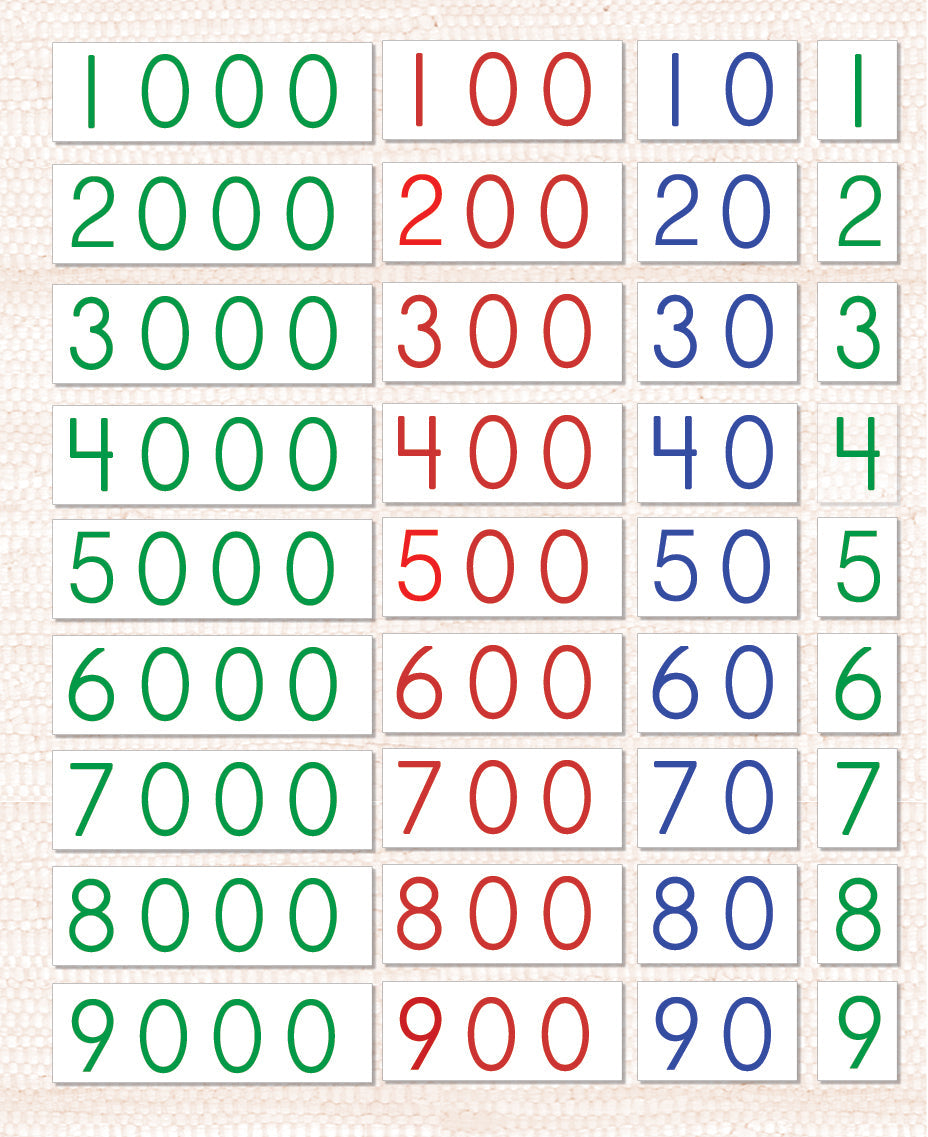
Decimal System

• Beads (concrete)
• Cards (symbol)
• Beads & Cards (concrete & symbol)

Operations: Concrete

• Bank Game (concrete)
• Stamp Game (concrete, symbol, & writing)
• Dot Game (symbol & writing)

Enumeration 10 & Above

• 11-19 Beads (concrete)
• Teen Board (symbol)
• Teen Board with Beads (concrete & symbol)
• Ten Board with Beads (concrete & symbol)
• Linear Counting: 100 & 1,000 Chains (concrete & symbol)
• Skip Counting: Bead Cabinet (concrete & symbol)
• Hierarchy Materials (concrete & symbol)

Operations: Bridge

• Snake Games (concrete)
• Multiplication bead layout (concrete)
• Strip Boards (concrete, symbol, & writing)
• Multiplication bead board (concrete & writing)
• Unit division board (concrete & writing)

Memorization

• Finger charts (concrete, symbol & writing)
• Control charts (symbol & control of error)
• Blind charts (symbol, writing, & control of error)

Abstraction

• Division with racks and tubes
• Small Bead Frame
• Large Bead Frame
• Decinomial cube

Lesson Plans & Resources

Be sure to practice your presentation alone or with a friend many times before presenting any materials to a child... you want your presentation to be flawless, precise, consistent/repeatable, and completely free from distractions so that the child can focus on, connect with, and learn from the materials.  Each lesson is like a choreographed dance that you memorize the movements for. Your performance of the movements must be precise but your interactions with an individual child will of course vary somewhat as you adapt to meet the needs of the moment. This flexibility amidst precision is the true art of guiding children. 

• Montessori Math Overview:  a PDF of a photo presentation of some of the main math materials in use in my old 3-6+ classroom (faces are obscured to protect anonymity of my students). I use it when I try to help non-Montessorian's gain some insight into the incredible intelligence of the concrete Montessori Math materials. 

• Math Material Organization, Scope & Sequence

• Math Equation Cards

• Math Equation Cards Lesson Plan

• Download our Math Equation Master on our Materials to Make page

• Just so you can see the quality of our digital files, we're offering you a free PDF of the Multiplication Bead Board. Use this to make your own board for remote/at-home learning or to print out the multiplication equation slips to replenish your stock. 
• Dot Game Lesson Plan
• This is a PDF of pages are straight from my AMI Montessori Album. They present basic information on how to present the dot game. If you are not a trained Montessorian, always seek the aid of one who is before presenting materials to the child. (Download Dot Game paper on our Materials to Make page.)